The circuit elements which are described in Application Note AN-EIS-003 can be combined in series and in parallel to build equivalent circuit models which can then be used to model the various phenomena occurring at the electrochemical interface.
This seven-part series introduces EIS and covers basic theory, experimental setups, common equivalent circuits used for fitting data, and tips for improving the quality of the measured data and fitting. This Application Note (part 4) provides example equivalent circuit models produced from the elements discussed in part 3. These models are among the most commonly encountered within electrochemistry research. The associated Nyquist plot is also shown for each model discussed.
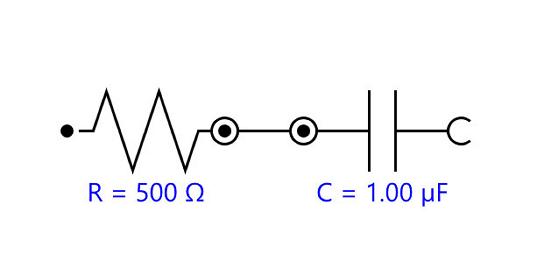
Model 1 (Figure 1) can be used, for example, to model a metal with an undamaged high-impedance coating. It can also be used to determine the double layer capacitance of a system if no faradaic reaction is occurring at the DC potential where it is measured. Here, the value of R gives the resistance of the electrolyte, and the value of C gives the coating capacitance.
In Figure 2, the resulting Nyquist plot is shown. It consists of a straight (capacitor) line at a value on the x-axis that corresponds to the resistance of the resistor in the circuit.
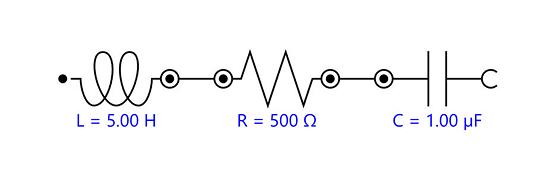
Model 2 (Figure 3) could be used to model the response of a supercapacitor. Here, the value of R gives the internal resistance of the supercapacitor, and the value of C gives the capacitance value of the supercapacitor, while L can be attributed to the cable’s inductance.
In Figure 4, the resulting Nyquist plot is shown. As introduced in the preceding Application Note (AN-EIS-003), this type of induction produces negative values for the imaginary impedance in the high frequency region of the plot.
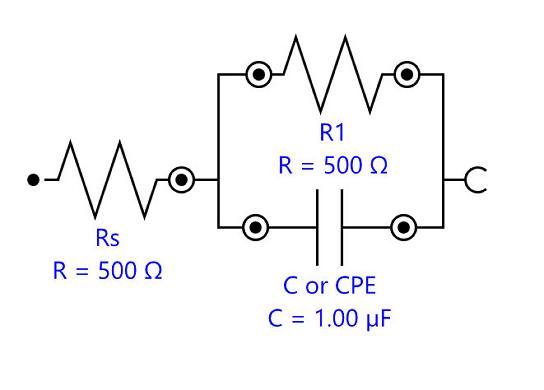
The Randles circuit (Figure 5) is one of the simplest and most common models for a three-electrode cell. It includes a solution resistance (RΩ), either a double layer capacitor C or a Constant Phase Element (CPE), and a generic element for the faradaic impedance, due to the electrochemical processes. In the simplest case of the Randles circuit, the faradaic impedance is solely the charge-transfer resistance Rct. It is often the starting point for other more complex models.
In Figure 6, a typical Nyquist plot resulting from the equivalent circuit of Model 3 is shown. The blue dots represent the Nyquist plot of the equivalent circuit with the capacitor, while the red dots represent the Nyquist plot of the equivalent circuit with a CPE (n = 0.8). The general recommendation is usually to use a CPE to account for any non-ideality in the capacitance of the system. Of course, there are exceptions to this. It is important to remember to use the circuit which makes the most sense in reality as opposed to the one that gives the best mathematical fit (see more in AN-EIS-005).
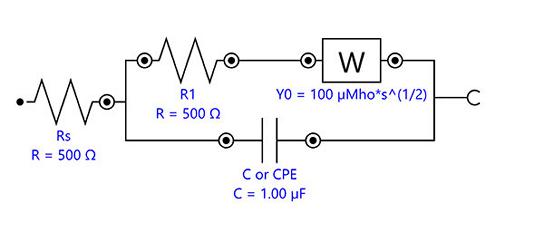
The Randles circuit can be easily modified to also describe electrode processes where both kinetics and diffusion play a role (Figure 7). Model 4 includes a solution resistance (RΩ), a double layer capacitor C (or a CPE), the charge-transfer resistance, and a Warburg element, ZWAR, which contains information about the diffusion coefficient for a species undergoing semi-infinite diffusion. Read AN-EIS-003 for more information about the Warburg element.
In Figure 8, a typical Nyquist plot resulting from the equivalent circuit in Model 4 is shown.
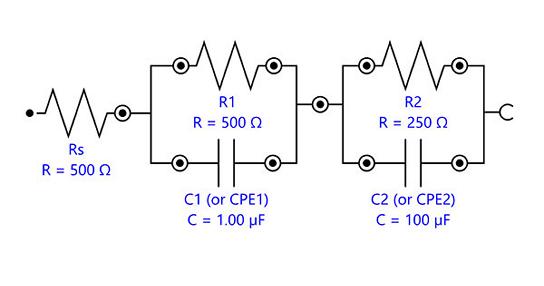
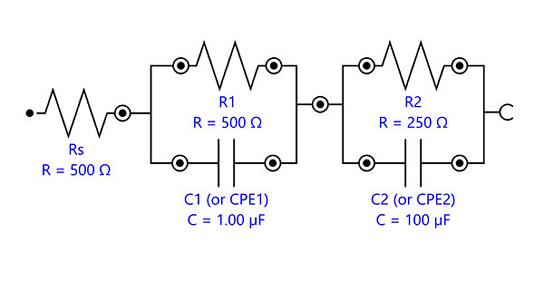
Model 5 can be used, for example, to model the response of batteries (Figure 9) or EC reactions involving adsorbed species (in this case, C2 or CPE2 is referred to as the adsorption capacitance). Here, each R and C (or CPE) in parallel can represent one electrode of the battery.
In Figure 10, a typical Nyquist plot is shown resulting from the equivalent circuit in Model 5.
Model 6 (Figure 11) can be used, for example, to describe the impedance of an organic coating on a metal substrate in contact with an electrolyte.
In Figure 12, a typical Nyquist plot is shown resulting from the equivalent circuit in Model 6.
It is important to remember that that the equivalent circuit modelling is a method that aims to match a theoretical model of an electrochemical interface with an experimental set of data. Proper assignment of the circuit elements can only be performed when sufficient information about the chemical and electrochemical phenomena taking place at the interface is available. Moreover, it is important to keep in mind that several arrangements of circuit elements are possible for a given set of data, and that some equivalent circuits are mathematically identical. In other words, it is important to not rely solely on EIS, and a combination of techniques should be employed to completely and accurately characterize the electrochemical interface. This limitation of EIS is explained more in the next Application Note in the series (AN-EIS-005).
This Application Note shows how electrical elements can be arranged in order to build both simple and more complex equivalent circuits that can be used for fitting EIS data. The resulting Nyquist plots for all the equivalent circuits are shown as well.
 Share via email
Share via email
 Download PDF
Download PDF